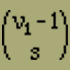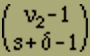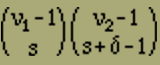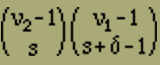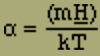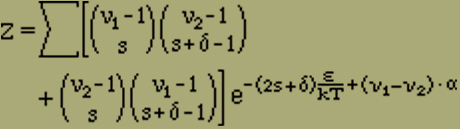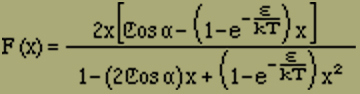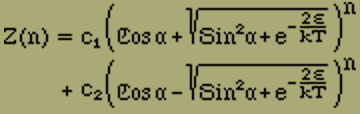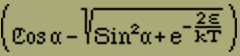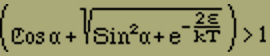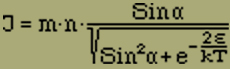Contribution to the

Theory of Ferromagnetism

1925

______________________________________________________________________________

2. The simple linear chain.

We want to apply these propositions to a model as simple as possible. We calculate the middle moment J of a linear magnet consisting of n elements. Each of these n elements should be able to take only two positions in which its moment m points in the ordered direction of the chain. We distinguish the two possible directions by the designations positive and negative, in short, we speak of positive and negative elements.

In order to calculate J, we consider an arrangement of the chain with ν₁ positive and ν₂ negative elements, such that

ν₁  +  ν₂  =  n.          (1)

For s positions, there will be negative elements inserted between the positive ones, i.e. on each of these s positions there is a group of negative elements which are connected to each other. For instance, for s = 3 we have

The chain begins at the left with a positive element and, depending on whether it ends on the right with a positive or negative element, a quantity δ will have the value 1 or 0. In the example above, we have δ = 1. Thus, the negative elements are, all in all, distributed on (s + δ) positions.

In the calculation we now make, at first it does not matter how many negative elements each of the (s + δ) groups contains, but only how large s itself is, the  number of gaps between the positive elements. One can choose these s gaps in

different ways. With each choice the ν₂ negative elements can be distri­buted in

ways 1) on (s + δ) positions. Altogether, the product

gives the number of arrangements described above. One obtains the number of corresponding arrangements beginning left with a negative element if one exchanges ν₁ and ν₂ in (2); it is therefore

We establish that the inner energy disappears when all elements are similarly oriented. But if similar poles of neighboring elements (i.e. positive and negative elements as designated above) interact on (2s + δ) positions (namely the 2s borders of the gaps and the edge of the remaining group on the right end), as is the case in the above considered arrangement, then such an arrangement should have the inner energy

(2s + δ) · ε     (ε > 0)

Since the moment of our arrangements is

(ν₁ - ν₂) m      (3)

their total energy in an outer magnetic field

(2s + δ)ε + (ν₂ - ν₁) (mH)      (3')

To abbreviate we set

where k = 1.37 · 10^-16, the Boltzmann constant, and T is the absolute temperature. To calculate the middle moment J, using equations (2), (2'), (3') and (4), we find the sum

Since in deriving Z from α, each member multiplies with (ν₁ - ν₂), namely with the moment belonging to it (3) except for the factor m, the middle moment can be obtained in the form

Equation (5) has to be summed up over ν₁ and ν₂ with the side condition (1) over s from 0 to infinity and for δ = 0.1 - for too large values of s the binomial coefficients disappear.

These summations are easy to perform if one considers the partition function Z(n) as a function of n and eliminates the side condition (1) by forming with the help of an arbitrary mathematical auxiliary variable x

where n in Z means ν₁ + ν₂. The calulation of F(x) is done by summing up from 0 to infinity over ν₁ and ν₂ independently of each other which can be done without difficulty with the help of the binomial theorem. When this has been done, we only have a geometrical series before us whose summation gives us

If one develops again this closed expression for F(x) by means of further continued fraction analysis for powers of x, one finds as coefficient of xⁿ

c₁ and c₂ are used here for abbreviations for two factors which do not play any part in the following; c₁ is for all values of magnitude 1. In Z one can neglect the factor

since this quantity is always < 1, while

and because n is a very large number. Since one can neglect the contribution of c₁ from

because n > 1, one finds, according to equation (6),

This function disappears for H = 0, i.e. α = 0; thus we find no appearances of hysteresis and no spontaneous magnetization. An increase of ε only causes a change in the form of the curve, so that saturation is reached more quickly and more completely.

Two reasons can be found for this behavior. Thus, if one considers H = 0, the probabilities as a function of the moment M, this curve has either two maxima symmetrically positioned around M = 0, and probably very steep, or, for reasons of symmetry, a single maximum at M = 0. From the result J = 0 of the average, one cannot, when the curve of the probabilities has two maxima, necessarily draw the conclusion that the middle moment, which is to be observed, disappears. It would be much more reasonable that the state of the magnet fluctuates for longer times, (compared to the length of the observation) around one of the two most probably equal moments which are opposite to each other and that thereby spontaneous magnetization could be observed. Unfortunately a simple calculation shows that the possibilities only have a simple maximum. Thus the larger expenditure of energy of the nonmagnetic state is compensated for by its extraordinarily large complexity.

As an analogous examination shows 2), nothing is changed in the result J = 0 for H = 0, when we include for the elements, besides the positive and negative positions, further orientations perpendicular thereto, or when we consider the mutual effect of second order elements next to the neighboring elements. 

――――――――――

1) One sees that one can distribute a number of m things in

ways on r positions or, what results in the same thing, that one can represent m as often as the sum of r whole numbers, if one represents m in the form 1 + 1 + . . . . + 1 and excludes (r - 1) from the (m - 1) plus signs. This is possible

times. For instance we have for m = 5 and r = 2

5 = (1) + (1 + 1 + 1 + 1) = (1 + 1) + (1 + 1 + 1)

= (1 + 1 + 1) + (1 + 1) = (1 + 1 + 1 + 1) + (1).  

2) Compare the original.